In this blog post I will show how an evanescent acoustic mode can be turned into a propagating mode via acoustic topology optimization.
For a circular cylindrical tube the so-called (0,1) mode has symmetry along the circumference of the tube, and one nodal line along the radius. Above a certain frequency, sometimes called the 'cut-off' frequency, othertimes (and what I prefer) 'cut-on' frequency, the mode can propagate. That means that given an infinitely long tube with a cross-section that never changes, and omitting any losses, the mode, if excited, will exists and an acoustic wave with the shape of the mode will propagate through the tube without ever being dampened. If however the mode is excited below its cut-on frequency, it cannot propagate, and the sound field is instead evanescent, extending a very short distance from the excitation plane with an exponential decay (I will make a blog post later that details this more).
Now, in previous posts, I have described how Topology Optimization can be used for acoustic design. Here, I try to apply the technique for converting an evanescent wave into a propagation wave. I use a 2D Axisymmetry simulation for the acoustics and the optimization. In the figures, however, I have expanded the solution into 3D space for visualization purposes. The target is then a propagation wave, but in 2DAxi, the only wave below the (0,1) mode is the plane wave, (0,0), and this wave is always propagating, and so the objective function is to have the best match between the sound field at the output of the tube and a plane wave. As always, I am using COMSOL Multiphysics, as this is by far the most suited for these more specialized acoustics case IMO.
First, the setup without any topology optimization. The (0,1) mode is excited at the left input, and because the excitation is only slightly below the cut-on frequency of the mode, the field can extend fairly far into the tube. It is however noticeably dampened through the tube. If it didn't extend far, it would be difficult to define the optimization design domain, and so the example is constructed to better show off the general idea.
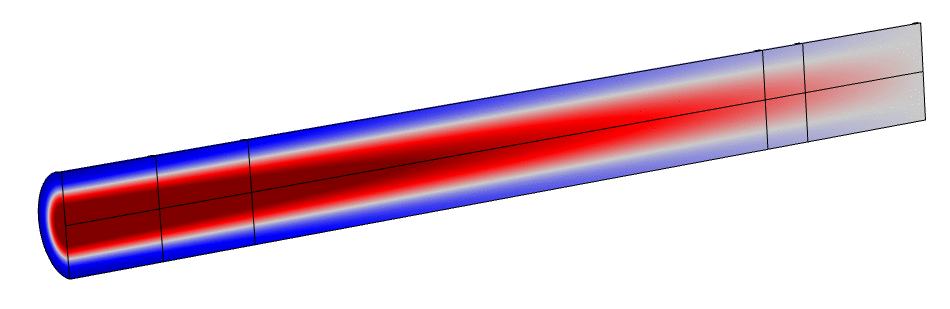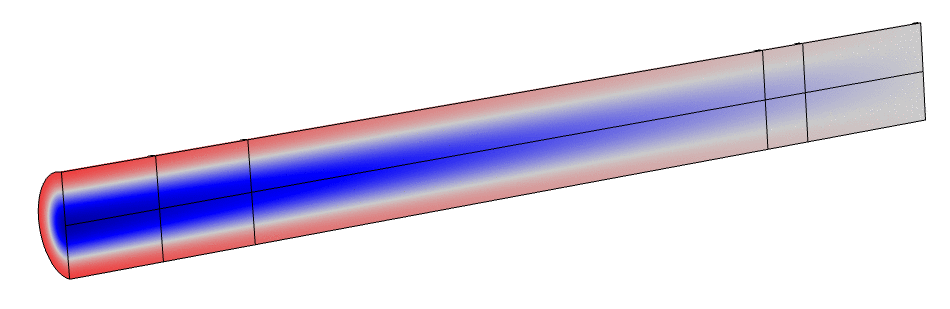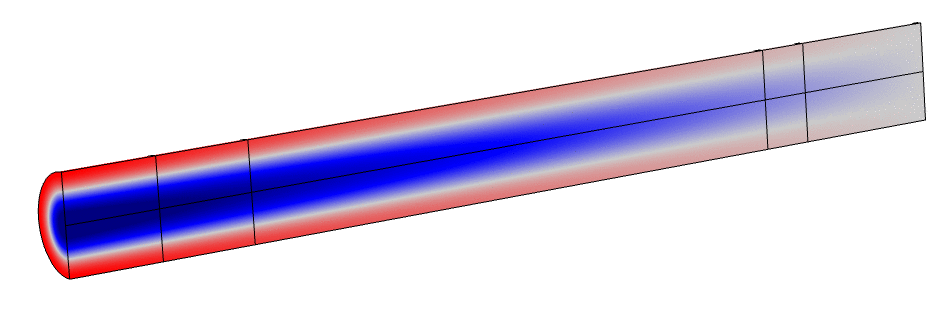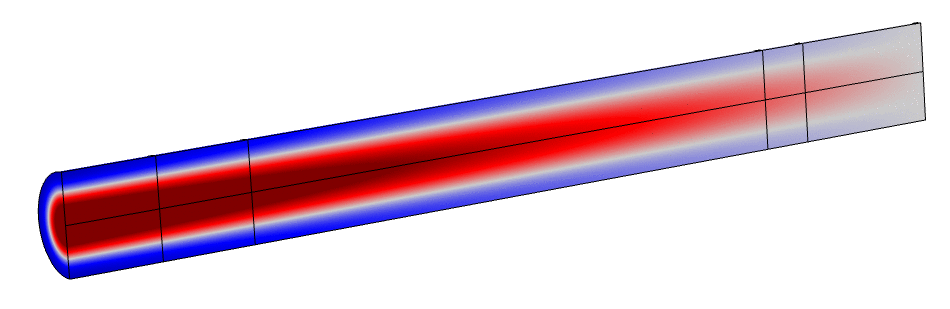
A certain section of the tube was assigned to be available for the topology optimization procedure, and so a binary design was found, that was in turn turned into a proper geometry. Exciting this new geometry with the same input, results in mode conversion, so that a plane wave propagates through the tube.
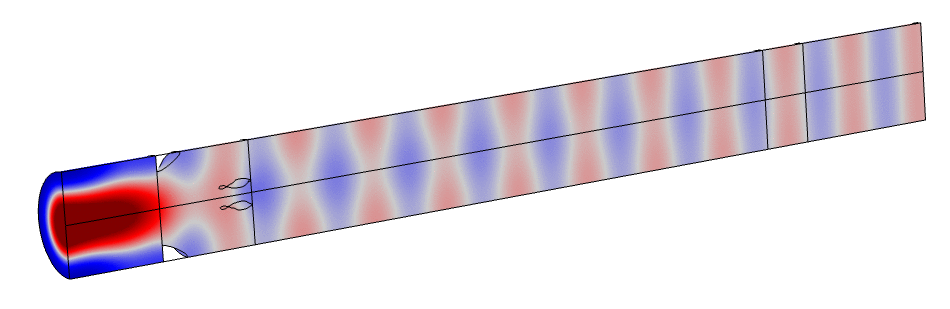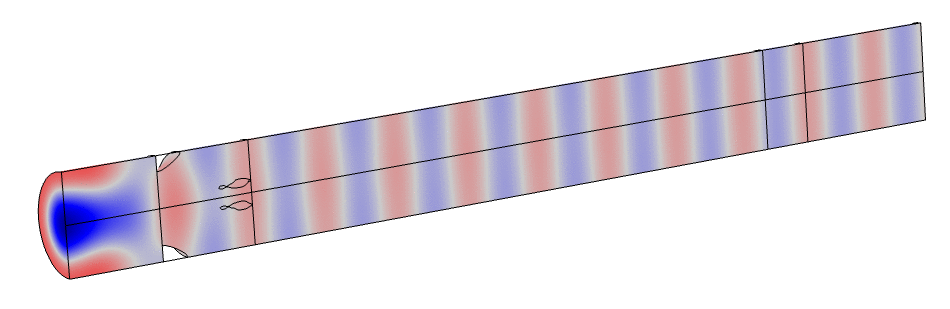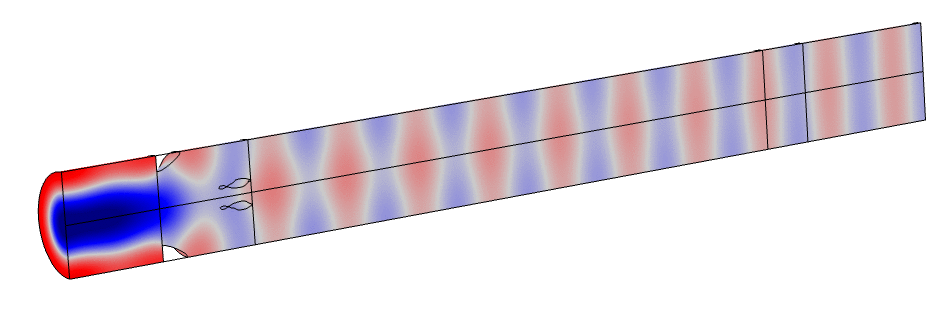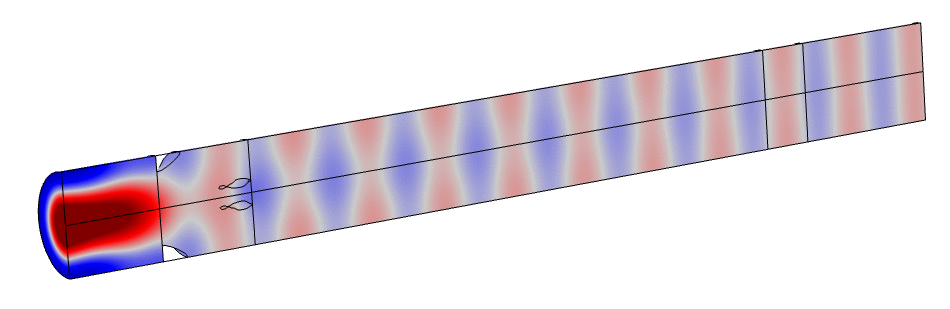
To me, this is really fascinating! Give this as task to an acoustics engineer with a lifetime of work experience, and he or she will probably have no idea how to tackle it. And yet, the software, having no concept of acoustics but only crunches number, gives a solution in a matter of minutes.
If topology optimization and/or acoustics is something that excites you, then hopefully some of my other blog posts are worth a read. Also, I will be presenting some of my research at the COMSOL Conference 2019 in Cambridge, and you are more than welcome to seek me out for a chat.
